Table of Contents
Mathematickal Arts
“To me the simple act of tying a knot is an adventure in unlimited space. A bit of string affords the dimensional latitude that is unique among the entities. For an uncomplicated strand is a palpable object that, for all practical purposes, possesses one dimension only. If we move a single strand out of the plane, interlacing at will, actual objects of beauty result in what is practically two dimensions; and if we choose to direct our strand out of this plane, another dimension is added which provides an opportunity that is limited only by the scope of our own imagery and the length of a ropemakers coil.” -The Book of Knots, Clifford W Ashley
Mathematickal arts workshop is organised by FoAM, as a part of Resilients (http://resilients.net) and Splinterfields (http://fo.am/splinterfields). The hypothesis of the workshop is that cultural resilience can be increased by (1) supporting a community of generalists able to connect disparate concepts and disciplines together, as well as (2) connecting traditional disciplines (such as mathematics and textile crafts) with contemporary and emerging technologies (such as computer programming or bioinformatics).
The workshop is designed and lead by Carole Collet, coming from textile education and sustainable design, together with Tim Boykett, a mathematician, artist and nautical enthusiast. The participants include a dozen people from a range of backgrounds: from textiles to free culture, graphic design to civil engineering, permaculture to lightweight structures, open source programming to cooking, with an even wider range of interests - origami to dough, fluffy dogs to algorithms.
Workshop leaders:
- Carole Collet (CSM Textile Futures, http://www.carolecollet.com/)
- Tim Boykett (Time's Up, http://www.algebra.uni-linz.ac.at/~tim/)
Workshop participants:
- Martaque
- Pieter Slock
- Eugénie Poste
- Wendy van Wynsberghe
- Fabian Feraux
- Stephanie Vilayphiou
- Miska Knapek
- Dave Griffiths
- Cocky Eek
Organisers/participants:
- Nik Gaffney
- Lina Kusaite
- Maja Kuzmanovic
Cook/participant:
- Annabel Meuleman
Day 1: 20110723
After the introductions to the workshop and its wider context of cultural resilience, the participants were invited to warm up by playing a human knot game - randomly joining all hands and trying to unknot to a loop or a twist without letting go (http://www.wikihow.com/Play-the-Human-Knot-Game). The theoretical session began with an overview of possible connections between mathematics and textile crafts. They talked about patterns and symmetries, knots and hyperbolic geometries, Jacquard looms, Indonesian double ikat and cellular automata.
Slides from the presentation: mathematickal_arts_2011.pdf
The presentation showed a broad range of possibilities that the workshop could unfold into, but its final direction depends on the participants and their interests. For example:
- group theory and different types of symmetries (http://en.wikipedia.org/wiki/Symmetry)
- tesselation, tiling and pleating (http://en.wikipedia.org/wiki/Tesselation)
- knotting, such as the Turk's Head (http://en.wikipedia.org/wiki/Turk%27s_head)
- braiding (http://en.wikipedia.org/wiki/Braids)
- short intro into continuous deformations (homotopy) of doughnuts into teacups in topology (http://en.wikipedia.org/wiki/Homotopy)
- weaving cellular automata (http://en.wikipedia.org/wiki/Cellular_automata)
- the hairy ball theorem (http://en.wikipedia.org/wiki/Hairy_ball_theorem)
Dissecting a Moebius strip
The first practical exercise took one of the seemingly simple mathematical phenomena - the Moebius strip, and attempted to intuitively predict what will happen when one begins cutting it. What will be the lengths, twists, knots? How would you explain what happen to someone who'd like to try it themselves?
After proceeding to cut, count and get entangled in strips of paper, the group came together to look at each other's notational experiments and diverse attempts to make sense of what was happening to the Moebius strip through a simple process of cutting - in words, diagrams and algoritms. One of the ways to make sense is to use mathematical reasoning - from a conjecture, through evidence and observation to a proof. For example:
Conjecture: When the length is twice as long it has twice as many twists; odd number of twists - you go around the loop twice, so you double the length and you double the twists - you can never get more than double the original length.
- odd number of twists –> 1/2 cut –> double length, double twist
- odd number of twists –> 1/3 cut –> double length double twist + same length, same twist
Evidence:
- 1 twist 1/2 cut = two pieces of twice the length, 2 twists
- 1 twist, 1/4 cut = 1 same length, 1 twist + 1 2 lengths, 2 twists
Observations:
- counting twists is hard
- when you bring patterns in your cuts, it gives you more headaches, but becomes a creative exercise
Summary of experiments (i.e. “what did we do”):
- We have taken a simple piece of material and done a series of experiments, taken notes and tried to make a summary of ideas in a working notation to help us remember what we've done, then sketched a proof to be able to repeat the experiment. The conclusion is that there are many different conjectures possible from a simple starting point.
- We got into trouble quite quickly (intuition was contrary to empirical evidence). Mathematician Trevor Evans said that if maths contributed anything to the world it was to put common sense in a box on a top shelf, just next to a box with nonsense (paraphrasing Tim). Maths keeps messing with our intuitions (common sense), in a repeatable way.
- We started with hands-on experiments, then moved on comparing and abstracting.
“Cutting a Môbius strip along the center line yields one long strip with two full twists in it, rather than two separate strips; the result is not a Möbius strip. This happens because the original strip only has one edge which is twice as long as the original strip. Cutting creates a second independent edge, half of which was on each side of the scissors. Cutting this new, longer, strip down the middle creates two strips wound around each other, each with two full twists.” http://en.wikipedia.org/wiki/M%C3%B6bius_strip
On hyperbolic geometry
Example of non-euclidean geometry challenging Euclid's parallel postulate:
- Euclid's geometry - flat surfaces, parallel lines never meet
- Circular geometry - there is always one point where two lines will meet
- Hyperbolic geometry - there are at least two parallel lines diverging
(More: http://en.wikipedia.org/wiki/Hyperbolic_geometry)
After a short demonstration of FoAM's stitched hyperbolic surfaces (http://www.flickr.com/photos/foam/sets/72157626527782401/), the participants had a quick crocheting tutorial and made their first attempts at crocheting hyperbolic surfaces (http://www.math.cornell.edu/~dwh/papers/crochet/crochet.html) in wool and rope.
In addition to stitching and knotting, folding flat square pieces of paper (i.e. origami) was a third technique used at the workshop to explore hyperbolic geometry. The participants began with single hyperbolic paraboloids (http://erikdemaine.org/hypar/), and branched out into origami tesselations, such as the “water bomb” (http://www.youtube.com/watch?v=VXIVHjws15U)
Movie suggestion:
- Between the folds (http://www.imdb.com/title/tt1253565/)
Software: Computational origami
- treemaker origami http://www.langorigami.com/science/treemaker/treemaker5.php4,
- anarchiste origami > http://www.le-crimp.org/spip.php?page=documents&id_article=11
- http://ordigami.net (artist Etienne Cliquet)
References to practical exercises:
- Cutting Moebius strips (how to: http://www.exo.net/~pauld/activities/mobius/mobiusdissection.html)
- Crocheting hyperbolic surfaces (http://bit.ly/pNd1iN)
- Using origami folding to create hyperbolic paraboloids (http://erikdemaine.org/hypar/hypar_folding_150.gif)
Ideas for day 2: Macramé to find out about different knot theories. Japanese bondage technique to avoid knots, installation with ropes. Remixing folds and knots, weaving the space, turning scribbles into weaves, weaves into knots…
Day 2: 20110724
Pieter - one of the participants at the workshop - recently graduated by developing software to parametrically design foldable architecture. He demonstrated two models of origami-like architectural structures, that he used to test his software. The structures begin as a flat plane, with a textile sandwiched between two layers of wooden panels, combined in such a way that when lifted up in a third dimension, the shape will form folds through mountains and valleys held together by waterproof textile 'hinges'. The result is an organic, seemingly fragile, but robust and adaptive form - inspiring for people interested in making resilient nomadic shelters
“An organism is so complex a thing, and growth so complex a phenomenon, that for growth to be so uniform and constant in all the parts as to keep the whole shape unchanged would indeed be an unlikely and an unusual circumstance. Rates vary, proportions change, and the whole configuration alters accordingly.” - D'Arcy Wentworth Thompson
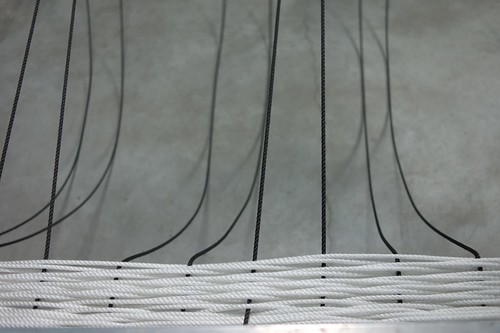
Spatial Weaving
The first exercise was an oversized demonstration of weaving a geometrical pattern using a black and white rope. The participants tied a loop of a weft around their waste and followed instructions to lift or lower their ropes, so the warp could pass through them, creating a woven pattern. After a short period of time, it became physically apparent why Jacquard designed an automated system for weaving complex patterns (http://en.wikipedia.org/wiki/Jacquard_weaving). A discussion ensued about different ways in which instructions could be given to each individual to create an algoritm of the weaving:
- each individual could be given a set of instructions, e.g. action 1: lower left raise right rope, action 2 - lift both ropes, action 3 - lower both ropes. Follow the sequence 1322331212123
- the instructions could be relative to their immediate neighbours (e.g mirror your neighbour)
- the instructions could be relative to the previous action (stay/change )
- …
Groups and repeats
From weaving to printing, the participants got their heads around group theory and making patterns for printing textiles, by exploring symmetry (http://en.wikipedia.org/wiki/Symmetry_group) and frieze groups (http://nrich.maths.org/1349) on paper. They compared the mathematical explanations with the less formulaic descriptions of creating repeated geometric patterns in textiles and texture design for games.
- how to make a seamless pattern using square cuts: http://okadadesign.blogspot.com/2010/10/pattern-repeat.html
Knotted dough
Bugnes, sweet French dough fritters are a wonderful way to explore knots, strips, planes, weaves and other forms in action. Stretching the dough and creating the form, then dipping it in boiling oil shows what happens when the form is subjected to a continuous transformation - a raw tea-cup becomes a fried doughnut, for example. The results were deliciously sweet and greasy afternoon snacks, happily eaten during the afternoon break.
Traditional Bugnes recipe: http://www.recette-dessert.com/bugnes-r307.htm
Complicating knots
Once the simple dough knots were digested, the group proceeded to complicate the matter with braiding the Turk's Head (http://www.animatedknots.com/turkshead/index.php), in synthetic and hemp rope, in a range of colours and sizes.
Suspensions
Knots are often used to hold things in place. How about using knots to hold things in space, in mid-air?
Using ropes and knots a series of cubes and hyper-cubes was suspended in the space, seeing how to deform the shapes through tension.
Fabian explored elegant Japanese bondage (http://en.wikipedia.org/wiki/Japanese_bondage) systems to suspend objects in mid air, using no knots but twists of hemp rope.
Steaming origami fabric
Polyester organza is easy to pleat by simple applying heat using the iron, oven or steaming it in the pressure cooker
Heat-setting synthetic textiles: http://www.tandfonline.com/doi/abs/10.1080/17543266.2010.517569?journalCode=tfdt20).
Origami in textile: http://www.shadowfolds.com/
Day 3: 20110725
The third day of the workshop began with a recap of things learned and tried out the previous day. A parade of repeats, textile origami, knotted suspended cubes and other mathematical textiles was observed with interest.
To bring a bit of 'magic' to the session, the workshop leaders devised a 'fridge/espresso poetry' game, where the participants picked four words out of four cups and constructed a sentence to guide their day's experiments. The verses included “Binding adaptive symmetry pocket”, “Symbiotic stitch algebra soft failure”, “Entangle stretching threads abstraction” and more.
The last hours of Mathematickal Arts were reserved to deeper explore ideas discovered in the first two days, especially ideas that the participants find most applicable in their practice. The participants proceeded to develop their experiments in smaller groups: textile origami, knotted suspensions, netting tour-de-france, ascii weaving, parametric software explorations, to name a few.
The workshop leaders re-introduced the question of the workshop - whether bringing mathematics and textiles together increases the resilience of both practices. One hypothesis is that by combining the two, a better balance can be found between a heuristic design approach and learning by abstraction, as is more common in mathematics.
One of the examples was the experiment with suspending a cube made out of rope. T participants began making the sculpture by intuitively cutting and assembling pieces of rope, which used a lot of material and many knots. After using mathematics to understand the more optimal way of building the cube, much less rope was used and a different knot, that allowed for easy adjustments. This combination of techniques made the suspended cube more adaptive and in a way more resilient. Another example was that applying mathematics to textiles makes the craft of mathematics understandable and applicable to non-experts and therefore more embedded in common understanding.
As a possible future reunion, Carole suggested a visit to Calais to look at the complexity of lace looms (http://bit.ly/1gOTai).
Finally, Tim's last experiment was to create a Moebius Turks head, and the group felt that something's shifted in the universe when the knot was completed: http://narval.republika.pl/mobius.html
Workshop Outcomes
Tour de France fish net
Martaque designed a net based on historical tour de France scores. She mapped out the progress of individual riders and relationship with other riders, using them as netting points. Every point in the net was determined by the position of two riders in the netting. In this way it was possible to tangibly visualise the speed of different riders at various points in the race. Martaque will use the learnings from this experiment to develop a netted artwork using the results of 2011 Tour de France.
Suspensions
Fabian Feraux used the suspended cube as a geometrical shape to enhance the suspensions of humans in space. The cube functions as an etherial aquarium, that changes shape as it is tensed by the elevation of the human body into it. He explored how much weight one can put on what angle of the cube, looking at tension on attachment points. He experimented with Japanese bondage, where knots are only made at the beginning of a new rope. After that, he uses only twists to restrict and suspend a body or an object. In Japanese bondage, a strictly codified system, knots are considered offensive and ugly, in addition to the practical consideration that they take too long to untie if something goes wrong. During the workshop he learned about calculating tension points using mathematics, which helps to decide the parameters of the work. For suspending humans, it remains crucial to experientially get know the body shape and its endurance limits. Fabian will continue his collaboration with Nik Gaffney to refine the equations for making a larger cube with a person suspended from all four points. He is interested in exploring suspending other, more curved platonic solids. However he is aware of the complexity of an adjustable form - if you move one knot, everything moves. The precision becomes in this case even more crucial.
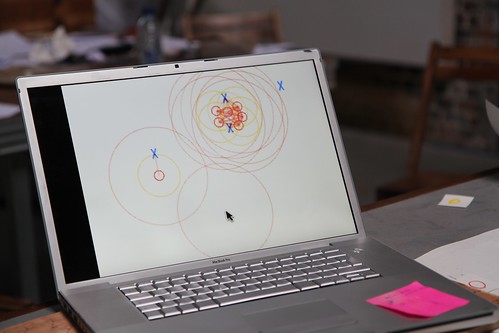
Simulation of elastic rope structures
Miska Knapek made a simulation of flexible structures, using physics of elastic bands. He creates attachment points and uses them as anchors, the program calculates the ideal distance for the elastics and keeps the structures in dynamic balance. Miska was inspired by varying elasticity of rope (hemp to nylon) and used parametric design to simulate the poetry of physical structures inherent in their emergent dynamics. The result was a small prototype of algoritmic graphics generated from varying relationships in a system, examining the properties of physically inspired pseudo/digital physics.
Textile origami
Cocky, Pieter, Wendy, Stephanie explored different origami patterns in textiles. One of the approaches was to sandwich polyester organza in-between a paper mould using paperclips. The textile and the mould were placed for 1 hr in the high pressure steamer. When the paper is taken away, the folds stay - polyester keeps the memory of the shape. A mix of polyester and metal proved not to be as successful. The pleats created in this way are permanent until the textile is heated to the same temperature. How strong the folds are depends on time, temperature and type of material.
The group looked at changing scales for origami textiles - using the same technique to design the textile for the body and textile for architecture. The challenge for the future is to see if larger structures can be made out of one material only (e.g. textile, rather than textile and wood). This has proven more challenging, but also more beautiful. The prototype made at the workshop were several paper modules of hyperbolic paraboloids, which were suspended (adding tension) to combine multiple modules. Once connected, the shape became multifaceted and organically curved. In this experiment, mathematics formed the shaping of the modules, which were individually and collectively responsible for the behaviour & form of the more complex structure. The group found it helpful to work with physical prototyping. If it had to be made on the computer it might have been too complicated to come to the same outcome, where the inside flows into the outside. The group finished by exploring different variations that can be made make by connecting only three modules. Working with a mathematician the finite number of possibilities could be calculated.
Wendy, Stephanie and Eugénie explored creating origami patterns by knotting textiles - building a vocabulary of dots, lines, squares, triangles - connecting mathematical geometry to the physical gestures of sowing and pleating. By combining different elements (symbols), new forms arise, it is like programming pleats. After the pleats are sown, they are ironed. The patterns are inspired by paper origami, but behave quite differently in the textile medium. By developing their own vocabulary of pleats, the group could begin to see cause and effect (if i do this, this happens), and came to their own conjunctures from which they could begin designing their own patterns, without premade manuals & models.
The group observed the difference in dimensionality between different materials - sown fabric produced 2 1/2 D, fabric and wood can be reversed from 2d to 3d and back again, while steamed organza patterns always stay 3D.
In addition to origami textiles, Wendy and Stephanie created crocheted hyperbolic sausages that they saw as inspirations for folding and origami books, both in paper and in textile.
Eugénie works together with dancers and is interested in making folds that can follow joints. Wendy will joing her from a Dance & Technology angle - thinking about making completely fabric sensors. The origami folds might help creating a third dimension in a fabric that moves on the body of a dancer. They will continue exploring different materials and colours to exaggerate the movement.
Ascii weaving
Dave Griffiths created little programs to create patterns that he would weave in textiles. The program starts with a rule and makes a sequence: e.g. when you encounter a yellow yarn, replace it with a sequence green, yellow, yellow, green. While Dave was weaving from command line, the computer was predicting what the pattern would look like in ascii code. The challenge was to see if the real weave matched with the ascii weave. The first prototype was made in plain weave, but adding more rules would help make something approaching a jacquard.
See more detail here:
Conclusion: what happens when you bring maths & textiles together?
A few reactions from participants
- The combination of textile and mathematics is very relevant - it clarifies some of the more abstract ideas in science and programming
- Learning how to make the turk's head was a revelation
- It was valuable to look at braiding more in detail and understand topological complexity in the real world (e.g.), rather than just abstractions
- Putting the code in textile - creating information inside the weaves, rather than on it. I'm reminded of threads and fibres as communication systems of the ancient Inkas: Description of a textile within a textile
- Self referential weaves as educational materials - showing what the rule set was that created them.
- Working with textiles informed me to make different forms in generative graphics
AHA moments
- memory shape fabrics
- calculation for the cube suspensions - understanding maths does help!
- understanding that variations are not endless and knowing that it is possible to know them all
- using a combination of fabric and wood for shelters, waterproof textile hinges is genious - Pieter's designs are an improvement on leaky hinges of Buckminster Fuller!
- i have more of an understanding about algorithmic design - reducing a method to something quite simple - short circuiting trial and error, finding productive directions more quickly and more clearly
- finding a new way of working - not only trial and error, not only abstractions, but something in betweens
- the saussage machine is a curiosity, it was great figuring out how it works
- 3 dimensionality of knots & hyperbolic planes (crocheting: repetition creates the planes) is a bit like recursive programming
- Force is not necessarily useful for hyperbolic crocheting
- This was a workshop with a high fun factor, so many things popped up suddenly and surprisingly
- the workshop made me specialise in being Martaque
- i hear a lot of music here, which is good!
- Annabel Meuleman's cooking is unbeatable:
notes & miscellanea
- book: origami tesselations by Eric Gjerde http://www.happyfolding.com/taxonomy/term/129
- blogpost on knitting and debian > http://www.capacitor.constantvzw.org/?p=612
- computational couture > http://www.kickstarter.com/projects/rhymeandreason/continuum-computational-couture?ref=live
- hexagonal paper dress > http://unlimiteddesigncontest.org/en/node/58/manual
Ideas:
- museums in Brussels and Gent
http://lace.lacefairy.com/Lace/International/Brussells.html
- Creating Sorting algorithms as per e.g. http://sortvis.org/ as textiles
- macrame as large scale lacemaking (is this true?)http://www.wikihow.com/Macrame
- 10mm braid rope - multiple colours, prefer nonpatterned rope so as to let the knot patters stand out
- should it be a Research Workshop rather than a doing learning workshop?
- Plan is that we do a series of smaller “exercises” over 1 (or 2?) days on various themes
- 2D Symmetries in fabrics, relation to the planar symmetry groups
- Use of symmetries, e.g. to be able to cut and still have appropriate pattern structures.
- Hiding symmetries (esp repetitions) in textiles
- Notational techniques in knitting and crochet, also weaving. relations to mathematical notations, procedural notations.
- Splicing in 3 and 4 core rope. Symmetry (in end splices) and symmetry breaking (in eye splicing), the two splicing techniques
- Parametrisation in textiles and sizes (related strongly to notation)
- Biology and mathematics?
- Knotting patterns that give emergent 3D structures (including hyperbolic_geometry): e.g. crochet→ Hyperbolic Reef (link needed).
- Given a pattern, what is the simplest algorithm to make it → Kolmogorov complexity
- Challenge: “How to learn some (nontrivial) mathematics through textiles”
- large scale 3D weaving - space filling cords.
- Museum visit at close of first day?
- Material Budget: around 700
- Dates: 3 days around the weekend of 23-25 July 2011
- Bridges Conference examples:
- Celtic knot patterns
- self similar knots
- weaving: see also html version here
- Moebius Strip knitting
- Question: are Celtic knot patterns related to Turk's Head knots?
Resources
- Lots of knots: http://www.realknots.com/
- Mathematical knitting: http://www.toroidalsnark.net/mathknit.html
- A nice article about knots, maths and textiles: http://www.yorktech.com/science/craig/Other/Knots.htm
- History of the Borromean Rings: http://www.liv.ac.uk/~spmr02/rings/
- Crafting by Concepts book; http://www.toroidalsnark.net/mkbook2.html
- The American Mathematical Society has a series of meetings: 2009,
misc:
- Brunnian rings: Nils Baas networks that unravel with a single cut.
Notes from dave
- requests: small frame loom + yarn